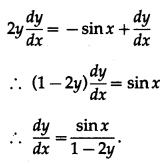Chapter 1 Differentiation Ex 1.3
Chapter 1 Differentiation Ex 1.3
Question 1.
Differentiate the following w.r.t. x:
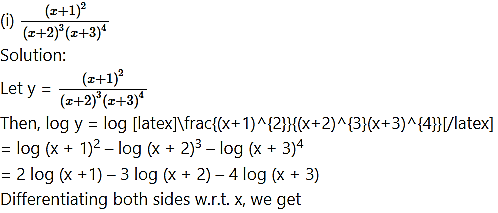
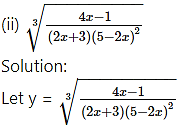
Then log y = log [latex]\sqrt[3]{\frac{4 x-1}{(2 x+3)(5-2 x)^{2}}}[/latex]
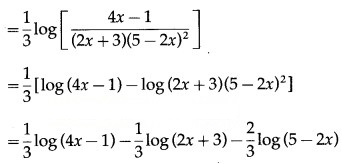
Differentiating both sides w.r.t. x, we get
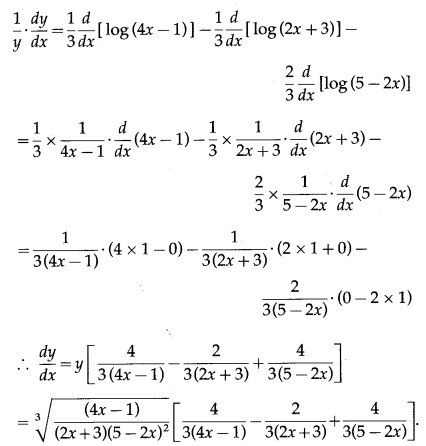
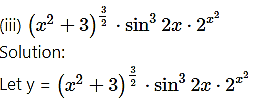
Then log y = log [latex]\left(x^{2}+3\right)^{\frac{3}{2}} \cdot \sin ^{3} 2 x \cdot 2^{x^{2}}[/latex]
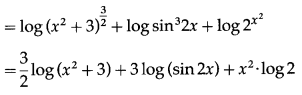
Differentiating both sides w.r.t. x, we get
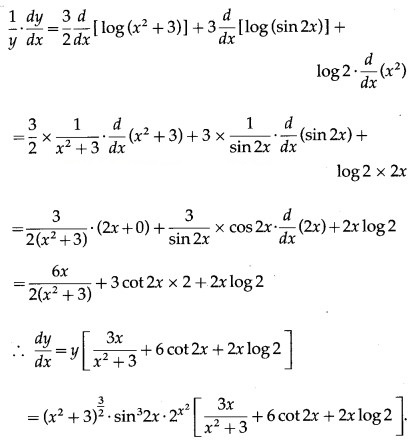
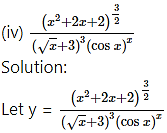
Then log y = log [latex]\frac{\left(x^{2}+2 x+2\right)^{\frac{3}{2}}}{(\sqrt{x}+3)^{3}(\cos x)^{x}}[/latex]
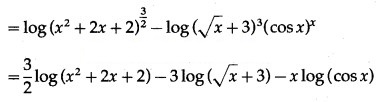
Differentiating both sides w.r.t. x, we get
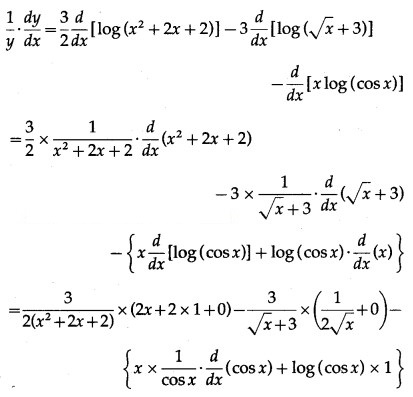
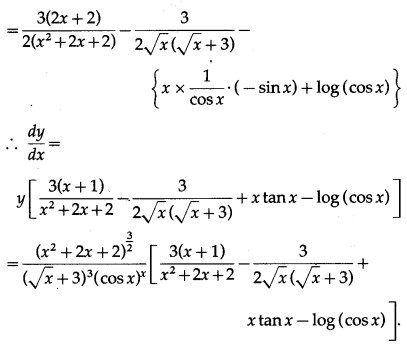
Then log y = log [latex]\frac{x^{5} \cdot \tan ^{3} 4 x}{\sin ^{2} 3 x}[/latex]
= log + log ta4x – log si3x
= 5 log x+ 3 log (tan 4x) – 2 log (sin 3x)
Differentiating both sides w.r.t. x, we get
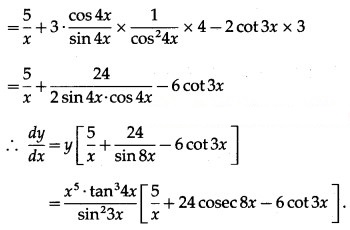
(vi)
Solution:
Let y =
Then log y = log () = ()(log x)
Differentiating both sides w.r.t. x, we get
(vii) (sin x
Solution:
Let y = (sin x
Then log y = log (sin x = x . log (sin x)
Differentiating both sides w.r.t. x, we get
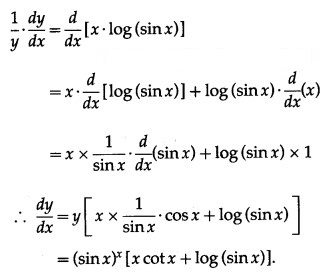
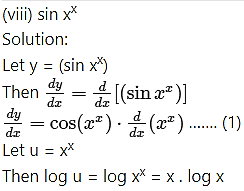
Differentiating both sides w.r.t. x, we get
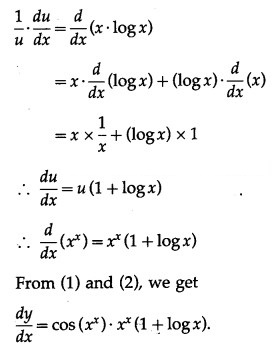
Question 2.
Differentiate the following w.r.t. x:
Differentiating both sides w.r.t. x, we get
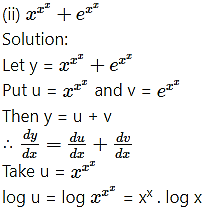
Differentiating both sides w.r.t. x, we get
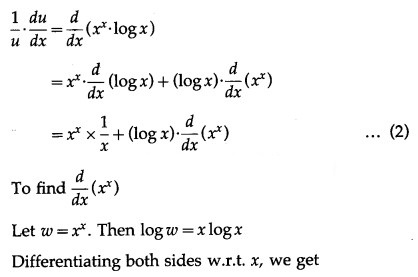
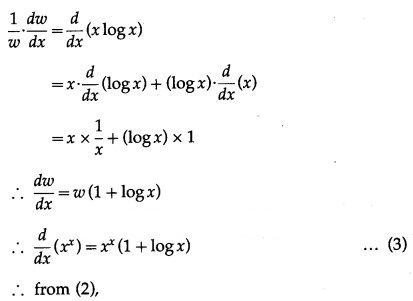
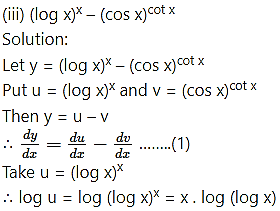
Differentiating both sides w.r.t. x, we get
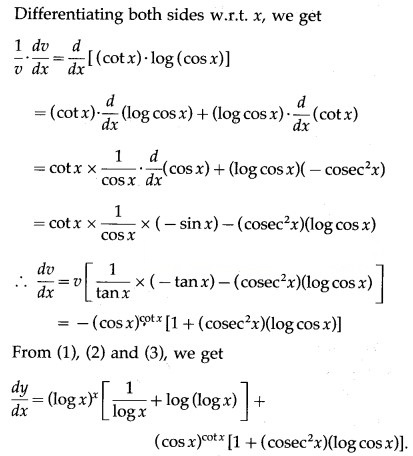
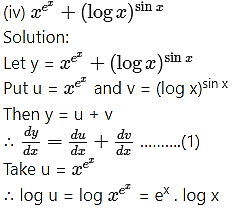
Differentiating both sides w.r.t. x, we get
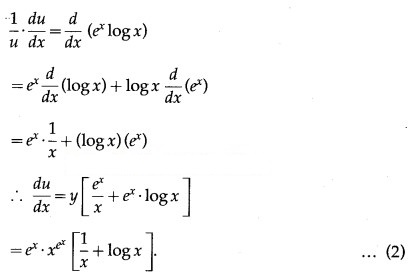
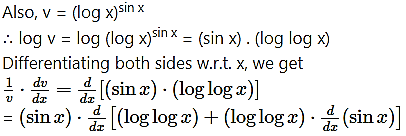
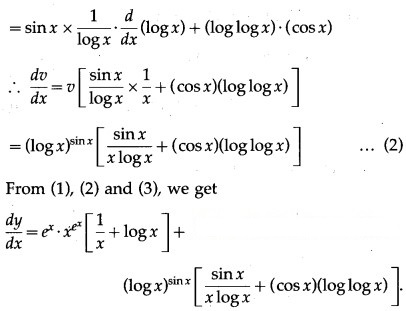
Differentiating both sides w.r.t. x, we get
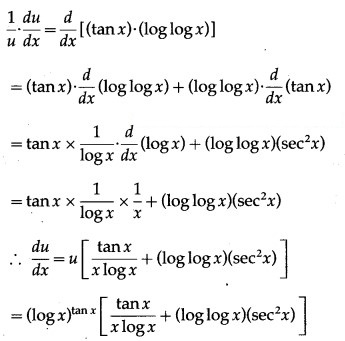
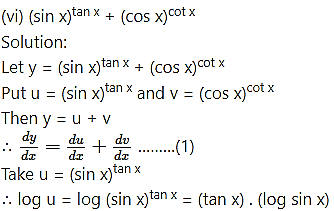
Differentiating both sides w.r.t. x, we get
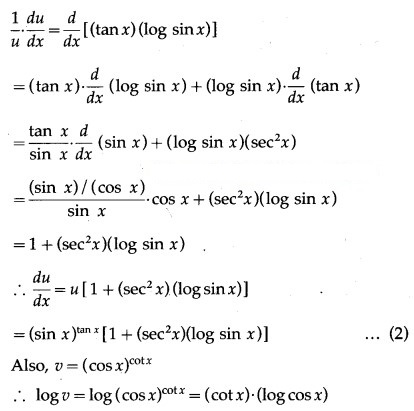
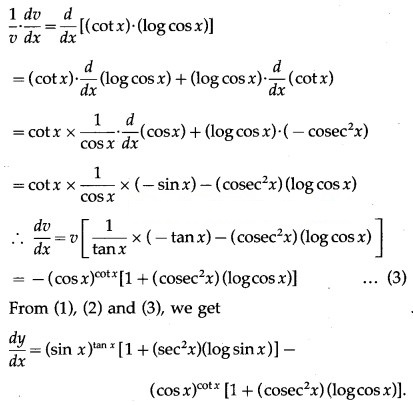
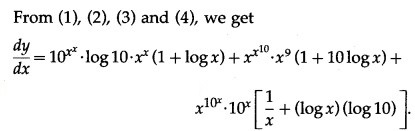
Differentiating both sides w.r.t. x, we get
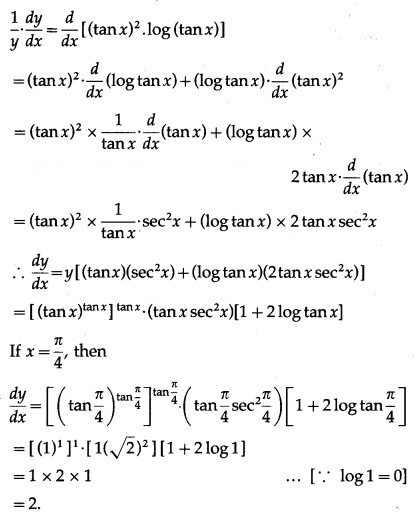
Question 3.
(i) √x + √y = √a
Solution:
√x + √y = √a
Differentiating both sides w.r.t. x, we get
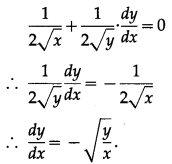
(ii) x√x + y√y = a√a
Solution:
x√x + y√y = a√a
Differentiating both sides w.r.t. x, we get
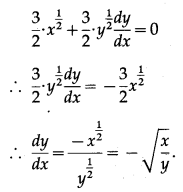
(iii) x + √xy + y = 1
Solution:
x + √xy + y = 1
Differentiating both sides w.r.t. x, we get
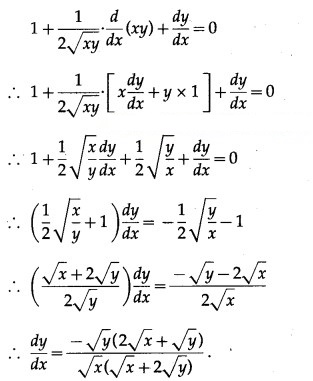
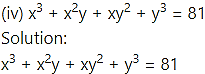
Differentiating both sides w.r.t. x, we get
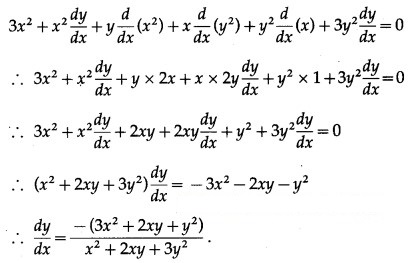
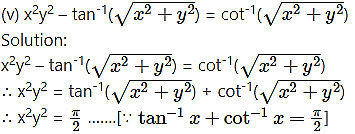
Differentiating both sides w.r.t. x, we get
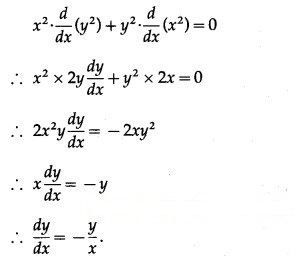
(vi)
Solution:
Differentiating both sides w.r.t. x, we get
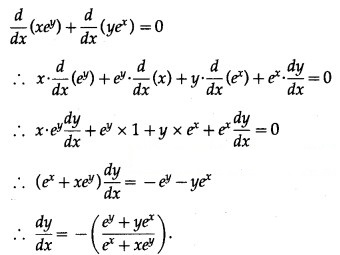
(vii) = cos (x – y)
Solution:
= cos (x – y)
Differentiating both sides w.r.t. x, we get
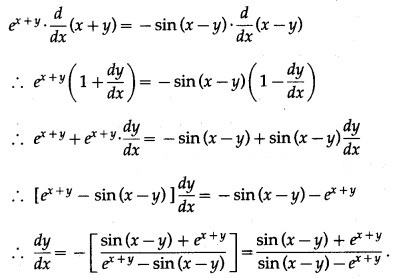
(viii) cos (xy) = x + y
Solution:
cos (xy) = x + y
Differentiating both sides w.r.t. x, we get
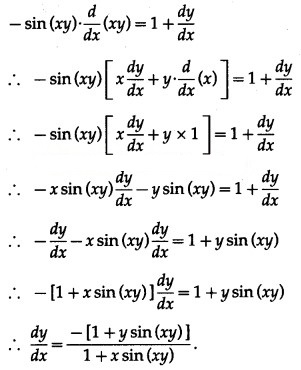
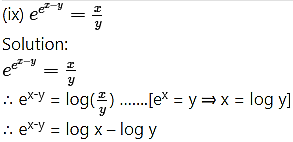
Differentiating both sides w.r.t. x, we get
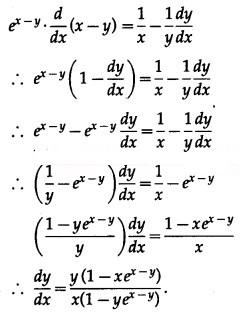
Question 4.
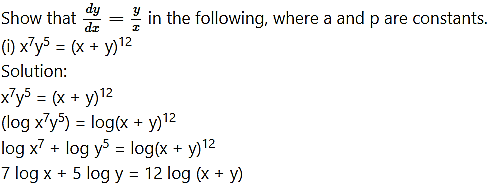
Differentiating both sides w.r.t. x, we get
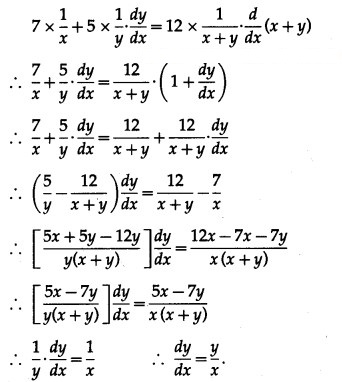
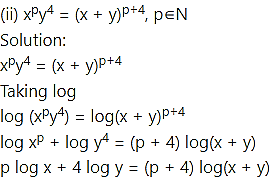
Differentiating both sides w.r.t. x, we get
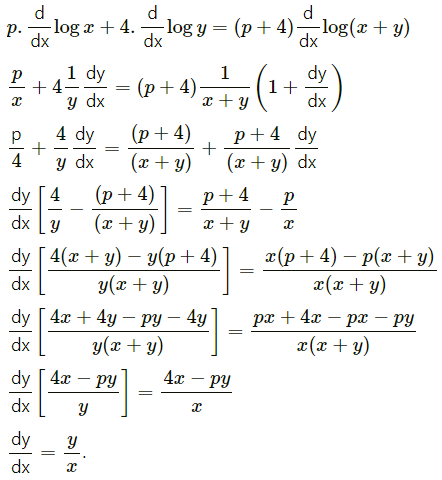
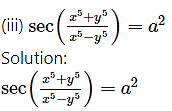
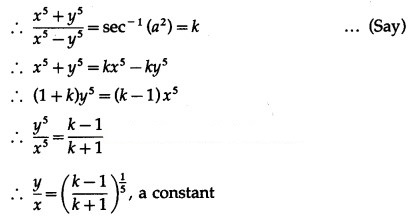
Differentiating both sides w.r.t. x, we get
Differentiating both sides w.r.t. x, we get
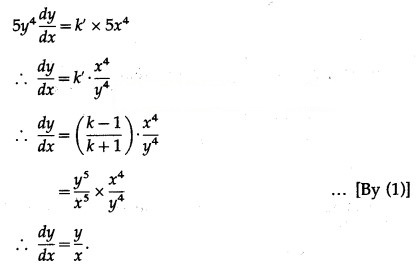
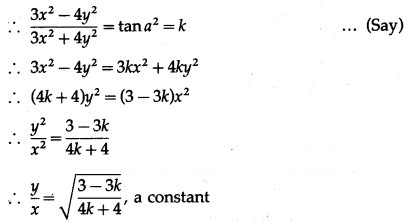
Differentiating both sides w.r.t. x, we get
Solution:
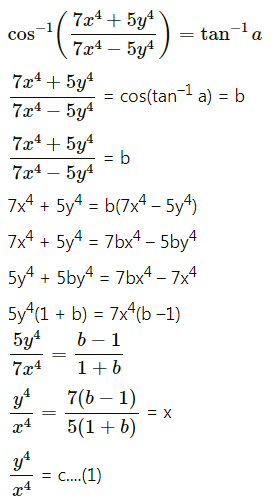
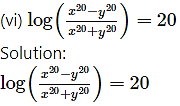
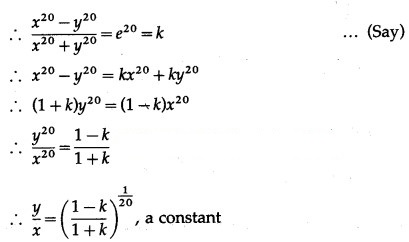
Differentiating both sides w.r.t. x, we get
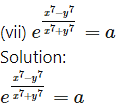
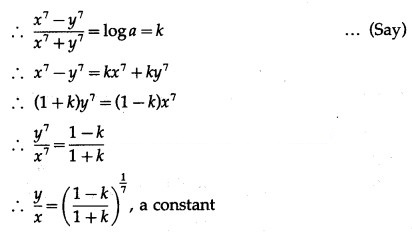
Differentiating both sides w.r.t. x, we get
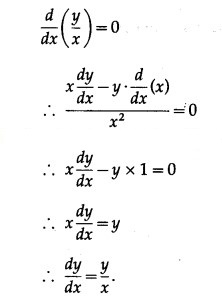
Solution:
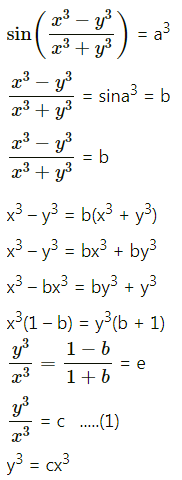
Differentiating both sides w.r.t. x, we get
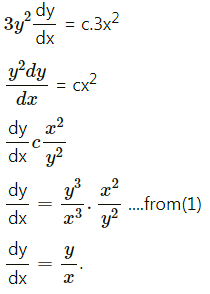
Question 5.
(i) If log (x + y) = log (xy) + p, where p is a constant, then prove that
Solution:
log (x + y) = log (xy) + p
∴ log (x + y) = log x + log y + p
Differentiating both sides w.r.t. x, we get
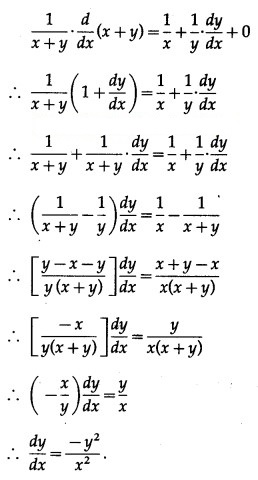
Solution:
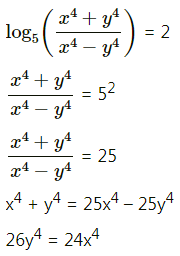
Differentiating both sides w.r.t. x, we get
Differentiating both sides w.r.t. x, we get
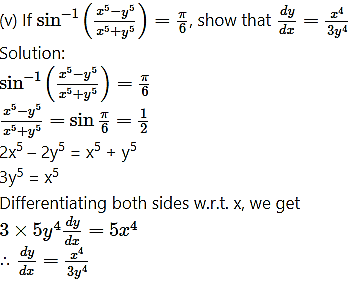
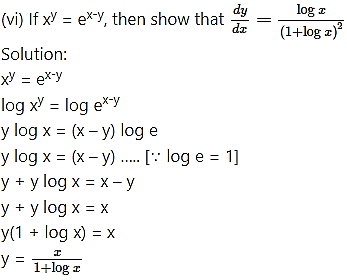
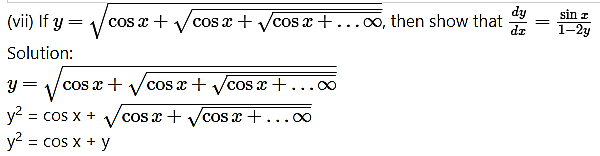
Differentiating both sides w.r.t. x, we get
