Blog

Chapter 3 Trigonometric Functions Miscellaneous Exercise 3

Chapter 3 Trigonometric Functions Miscellaneous Exercise 3

I) Select the correct option from the given alternatives.
Question 1.
The principal of solutions equation sinθ = −1/2 are ________.

Question 2.
The principal solution of equation cot θ = √3 ___________.

Solution:

Question 3.
The general solution of sec x = √2 is __________.

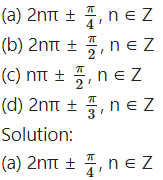
Question 4.
If cos pθ = cosqθ, p ≠ q rhen ________.

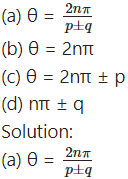
Question 5.
If polar co-ordinates of a point are (2,π/4) then its cartesian co-ordinates are ______.

Question 6.
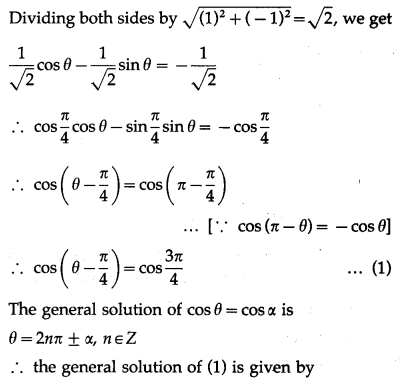
If √3 cosx – sin x = 1, then general value of x is _________.

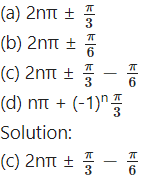
Question 7.
In ∆ABC if ∠A = 45°, ∠B = 60° then the ratio of its sides are _________.

Question 8.
In ∆ABC, if c² + a² – b² = ac, then ∠B = __________.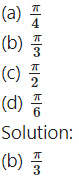

Question 9.
In ABC, ac cos B – bc cos A = ____________.
(a) a² – b²
(b) b² – c²
(c) c² – a²
(d) a² – b² – c²
Solution:
(a) a² – b²

Question 10.
If in a triangle, the are in A.P. and b : c = √3 : √2 then A is equal to __________.
(a) 30°
(b) 60°
(c) 75°
(d) 45°
Solution:
(c) 75°

Question 11.

Question 12.
The value of cot (tan^-1 2x + cot^-1 2x) is __________.
(a) 0
(b) 2x
(c) π + 2x
(d) π – 2x
Solution:
(a) 0

Question 13.


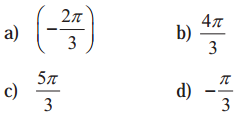
Solution:

Question 14.

Question 16.

Question 17.

Question 18.
The principal value branch of sec^-1 x is __________.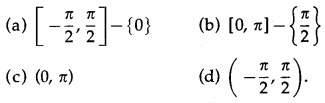

Solution:

Question 20.
If tan θ + tan 2θ + tan 3θ = tan θ∙tan 2θ∙tan 3θ, then the general value of the θ is _______.

Question 21.
If any ∆ABC, if a cos B = b cos A, then the triangle is ________.
(a) Equilateral triangle
(b) Isosceles triangle
(c) Scalene
(d) Right angled
Solution:
(b) Isosceles triangle

II: Solve the following
Question 1.
Find the principal solutions of the following equations :
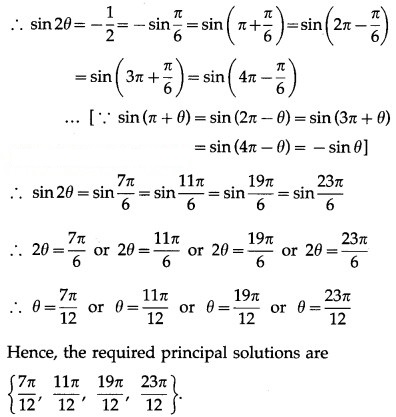
(i) sin2θ = −1/2
Solution:
sin2θ = −1/2
Since, θ ∈ (0, 2π), 2∈ ∈ (0, 4π)

(ii) tan3θ = -1
Solution:
Since, θ ∈ (0, 2π), 3∈ ∈ (0, 6π)

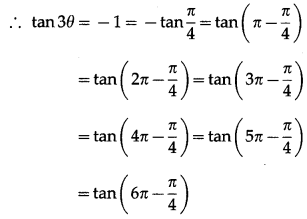
… [∵ tan(π – θ) = tan(2π – θ) = tan(3π – θ)
= tan (4π – θ) = tan (5π – θ) = tan (6π – θ) = -tan θ]

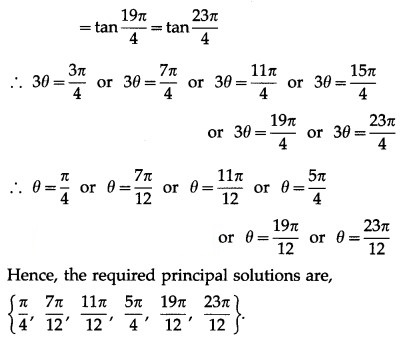
(iii) cotθ = 0
Solution:

Question 2.
Find the principal solutions of the following equations :

Solution:

(ii) tan5θ = -1
Solution:

(iii) cot2θ = 0
Solution:

Question 3.
Which of the following equations have no solutions ?
(i) cos 2θ = 1/3
Solution:
cos 2θ = 1/3
Since 1/3 ≤ cosθ ≤ 1 for any θ
cos2θ = 1/3 has solution

(ii) cos² θ = -1
Solution:
cos2θ = -1
This is not possible because cos2θ ≥ 0 for any θ.
∴ cos2θ = -1 does not have any solution.

(iii) 2 sinθ = 3
Solution:
2 sin θ = 3 ∴ sin θ = 3/2
This is not possible because -1 ≤ sin θ ≤ 1 for any θ.
∴ 2 sin θ = 3 does not have any solution.

(iv) 3 sin θ = 5
Solution:
3 sin θ = 5
∴ sin θ = 5/3
This is not possible because -1 ≤ sin θ ≤ 1 for any θ.
∴ 3 sin θ = 5 does not have any solution.

Question 4.
Find the general solutions of the following equations :

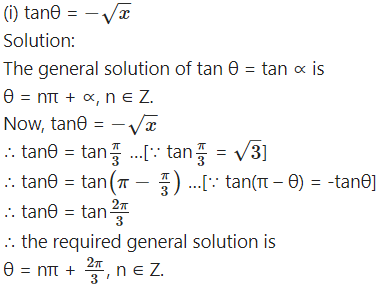
(ii) tan²θ = 3
Solution:

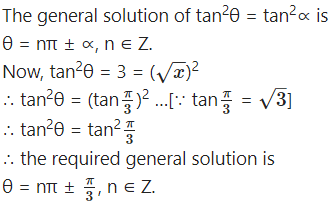
(iii) sin θ – cosθ = 1
Solution:
∴ cosθ – sin θ = -1

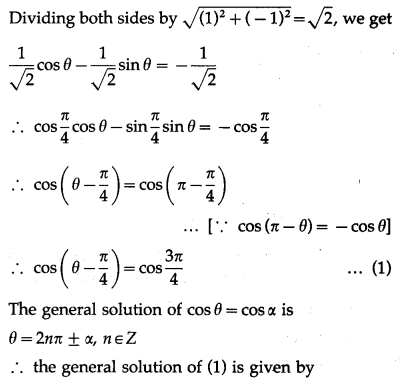
(iv) sin²θ – cos²θ = 1
Solution:
sin²θ – cos²θ = 1
∴ cos²θ – sin²θ = -1
∴ cos2θ = cosπ …(1)
The general solution of cos θ = cos ∝ is
θ = 2nπ ± ∝, n ∈ Z
∴ the general solution of (1) is given by
2θ = 2nπ ± π, n ∈ Z
∴ θ = nπ ± π2, n ∈ Z

Question 5.

Solution:
By the sine rule,

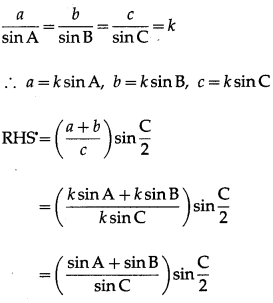
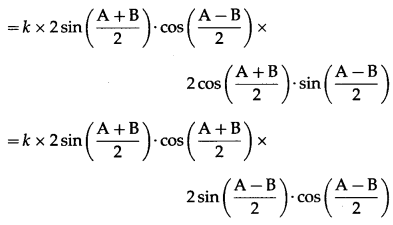
Question 6.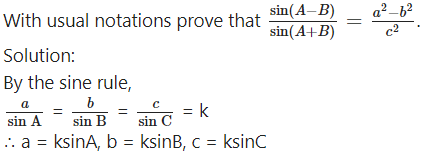

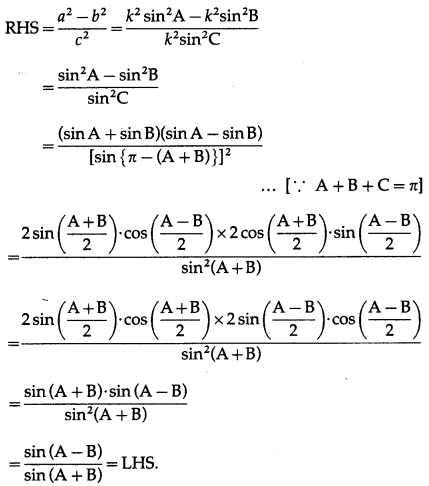
Question 7.

Question 8.
In ∆ABC if cosA = sin B – cos C then show that it is a right angled triangle.
Solution:
cos A= sin B – cos C
∴ cos A + cos C = sin B

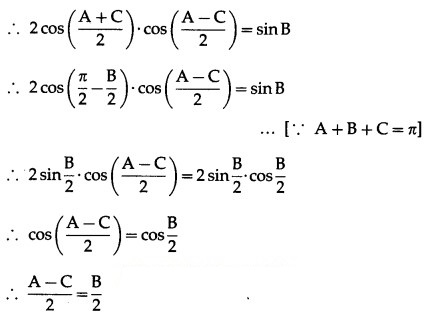
∴ A – C = B
∴ A = B + C
∴ A + B + C = 180° gives
A + A = 180°
∴ 2A = 180 ∴ A = 90°
∴ ∆ ABC is a rightangled triangle.

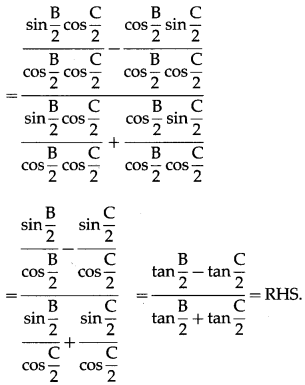
Question 9.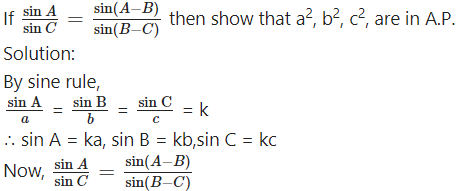

∴ sin [π – (B + C)] ∙ sin (B – C)
= sin [π – (A + B)]∙sin (A – B) … [∵ A + B + C = π]
∴ sin(B + C) ∙ sin(B – C) = sin (A + B) ∙ sin (A – B)
∴ sin²B – sin²C = sin²A – sin²B
∴ 2 sin²B = sin²A + sin²C
∴ 2k²b² = k²a² + k²c²
∴ 2b² = a² + c²
Hence, a², b², c² are in A.P.

Question 10.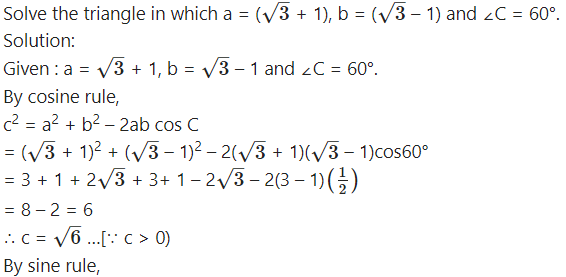


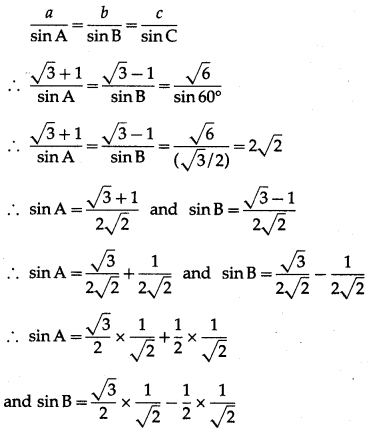
∴ sin A = sin 60° cos 45° + cos 60° sin 45°
and sin B = sin 60° cos 45° – cos 60° sin 45°
∴ sin A = sin (60° + 45°) – sin 105°
and sin B = sin (60° – 45°) = sin 15°
∴ A = 105° and B = 15°
Hence, A = 105°, B 15° and C = √6 units.

Question 11.
In ∆ABC prove the following :
(i) a sin A – b sin B = c sin (A – B)
Solution:
By sine rule,

∴ a = ksinA, b = ksinB, c = ksinC,
LHS = a sin A – b sinB
= ksinA∙sinA – ksinB∙sinB
= k (sin²A – sin²B)
= k (sin A + sin B)(sin A – sin B)

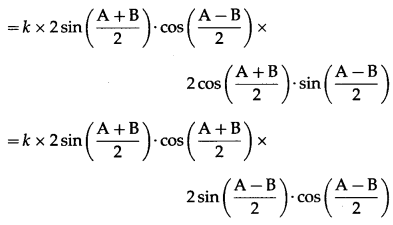
= k × sin (A + B) × sin (A – B)
= ksin(π – C)∙sin(A – B) … [∵ A + B + C = π]
= k sinC∙sin (A – B)
= c sin (A – B) = RHS.

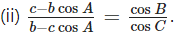
Solution: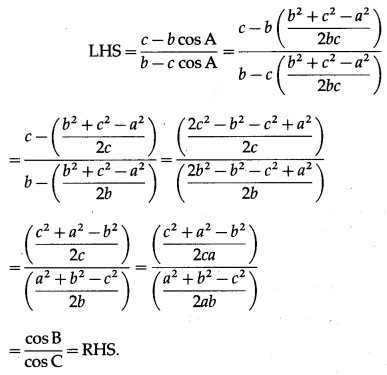

(iii) a² sin (B – C) = (b² – c²) sinA
Solution:
By sine rule,

∴ a = ksinA, b = ksinB, c = ksinC
RHS = (b² – c²) sin A
= (k²sin²B – k²sin²C)sin A
= k²(sin²B – sin²C) sin A
= k²(sin B + sin C)(sin B – sin C) sin A

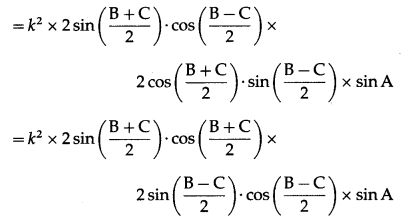
= k² × sin (B + C) × sin (B – C) × sin A
= k²∙sin(π – A)∙sin(B – C)∙sinA … [∵ A + B + C = π]
= k²sin A∙sin (B – C)∙sin A
= (k sin A)²∙sin (B – C)
= a²sin (B – C) = LHS.

(iv) ac cos B – bc cos A = (a² – b²).
Solution:

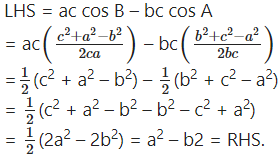
Solution:

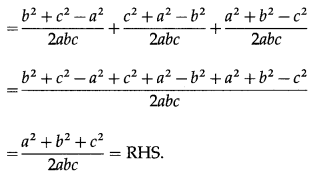
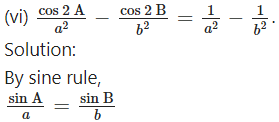
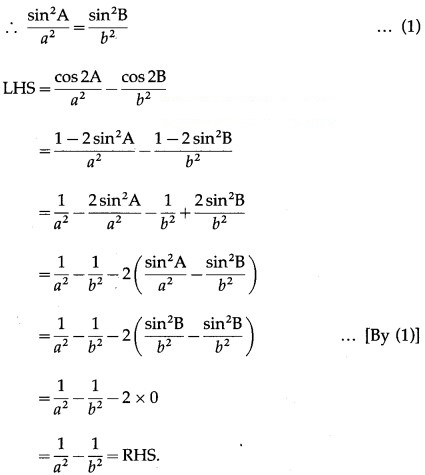
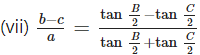
Solution:
By sine rule,

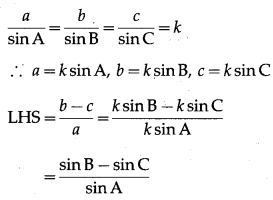
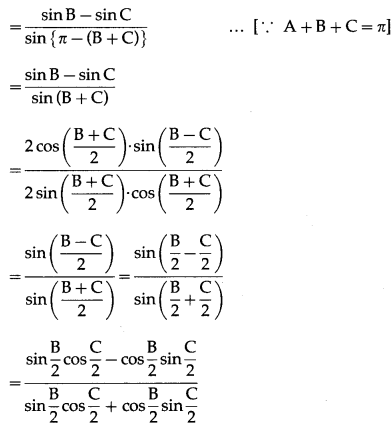
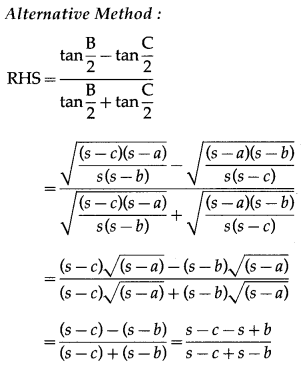
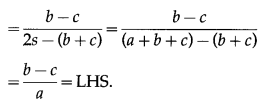
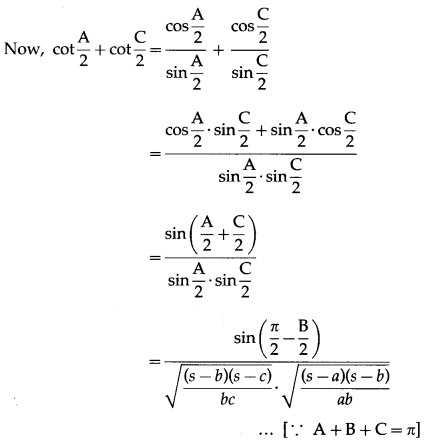
Question 12.

Solution:
a, b, c, are in A.P.
∴ 2b = a + c …(1)

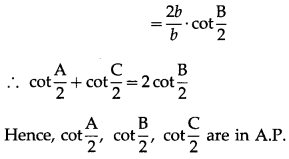
Question 13.

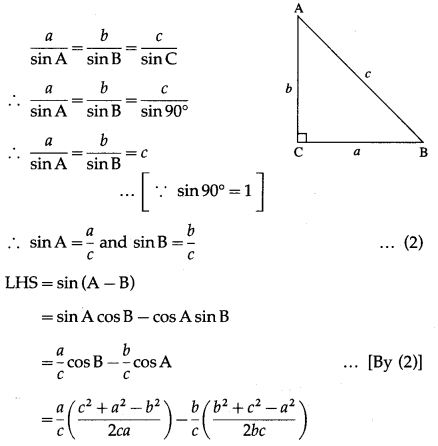
Solution:
In ∆ABC, if ∠C = 90º
∴ c² = a² + b² …(1)
By sine rule,

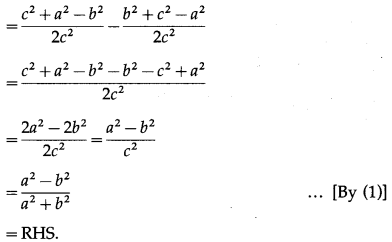
Question 14.

By sine rule,

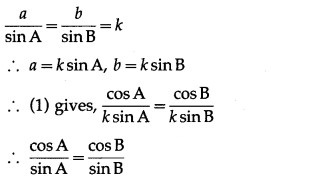
∴ sin A cos B = cos A sinB
∴ sinA cosB – cosA sinB = 0
∴ sin (A – B) = 0 = sin0
∴ A – B = 0 ∴ A = B
∴ the triangle is an isosceles triangle.

Question 15.
In ∆ABC if sin²A + sin²B = sin²C then prove that the triangle is a right angled triangle.
Question is modified
In ∆ABC if sin²A + sin²B = sin²C then show that the triangle is a right angled triangle.
Solution:
By sine rule,

∴ sin A = ka, sinB = kb, sin C = kc
∴ sin²A + sin²B = sin²C
∴ k²a² + k²b² = k²c²
∴ a² + b² = c²
∴ ∆ABC is a rightangled triangle, rightangled at C.

Question 16.
In ∆ABC prove that a²(cos²B – cos²C) + b²(cos²C – cos²A) + c²(cos²A – cos²B) = 0.
Solution:
By sine rule,

LHS = a²(cos²B – cos²C) + b²( cos²C – cos²A) + c²(cos²A – cos²B)
= k²sin²A [(1 – sin²B) – (1 – sin²C)] + k²sin²B [(1 – sin²C) – (1 – sin²A)] + k²sin²C[(1 – sin²A) – (1 – sin²B)]
= k²sin²A (sin²C – sin²B) + k²sin²B(sin²A – sin²C) + k²sin²C (sin²B – sin²A)
= k²(sin²A sin²C – sin²Asin²B + sin²A sin²B – sin²B sin²C + sin²B sin²C – sin²A sin²C)
= k²(0) = 0 = RHS.

Question 17.
With usual notations show that (c² – a² + b²) tan A = (a² – b² + c²) tan B = (b² – c² + a²) tan C.
Solution:
By sine rule,

∴ a = fksinA, b = ksinB, c = ksinC


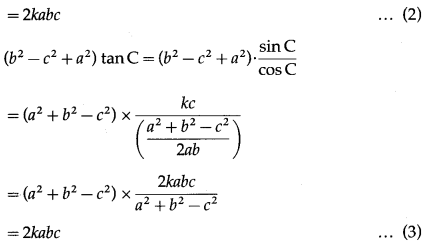
From (1), (2) and (3), we get
(c² – a² + b²) tan A = (a² – b² + c²) tan B
= (b² – c² + a²) tan C.

Question 18.


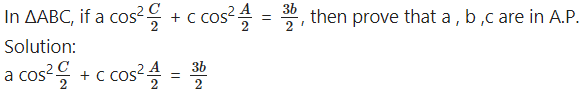
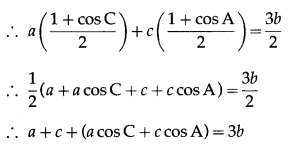
∴ a + c + b = 3b …[∵ a cos C + c cos A = b]
∴ a + c = 2b
Hence, a, b, c are in A.P.

Question 19.





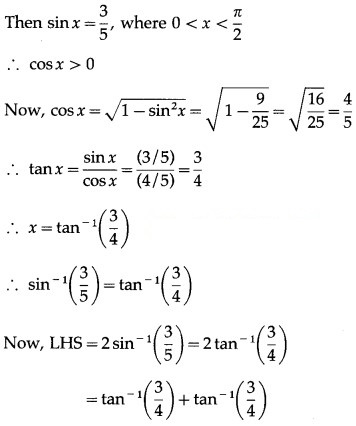
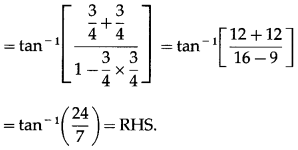
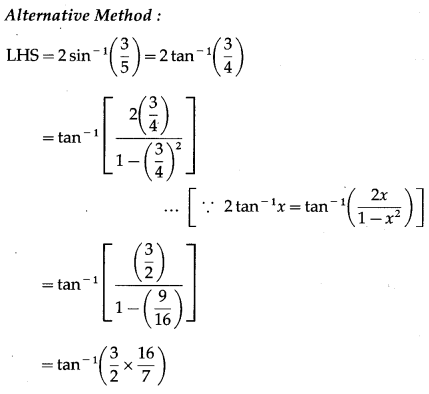
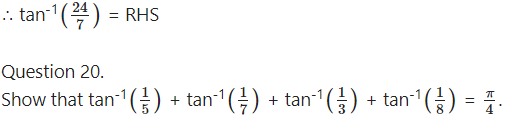
Solution:

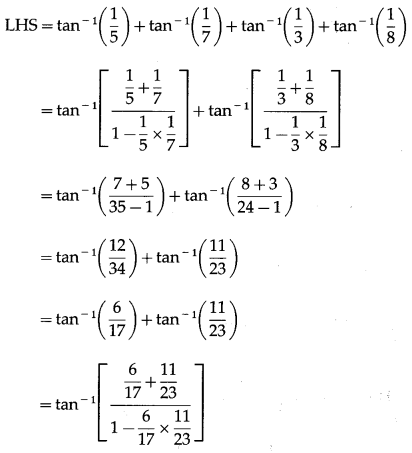
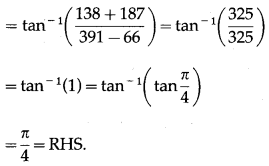
Question 21.

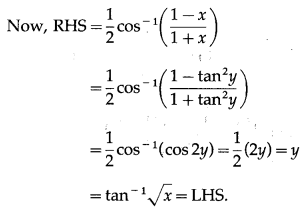
Question 22.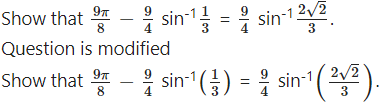

Solution:
We have to show that

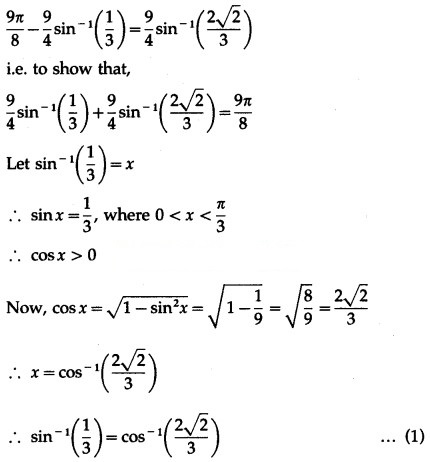
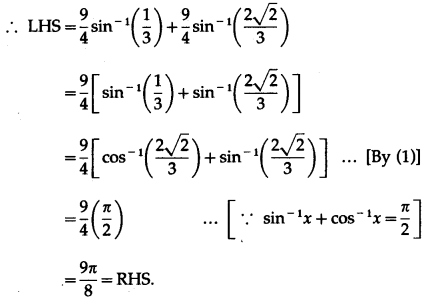
Question 23.
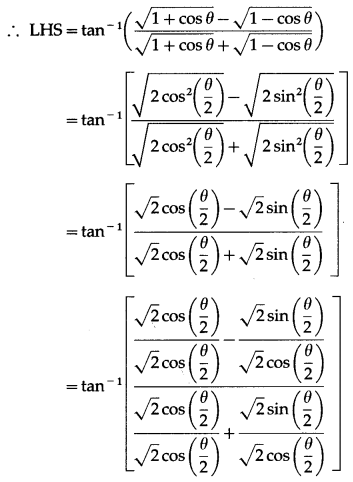
Show that

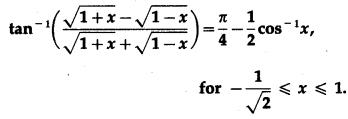
Solution:

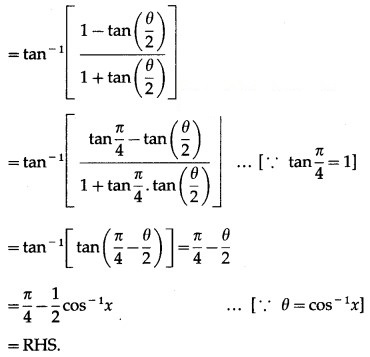
Question 24.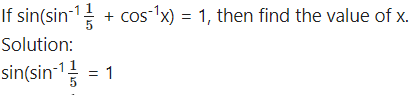

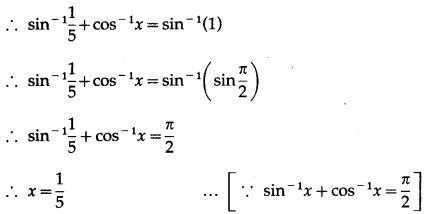
Question 25.
If tan^-1(x−1x−2) + tan^-1(x+1x+2) = π4 then find the value of x.
Solution:
tan^-1(x−1x−2) + tan^-1(x+1x+2) = π4

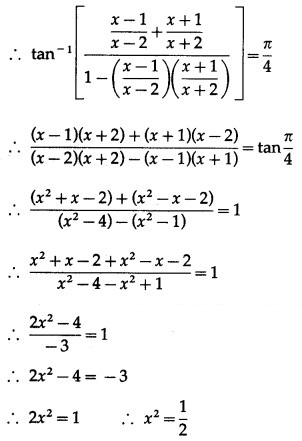
Question 26.
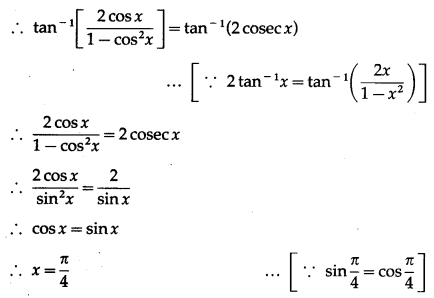
If 2 tan^-1(cos x ) = tan^-1(cosec x) then find the value of x.
Solution:
2 tan^-1(cos x ) = tan^-1(cosec x)

Question 27.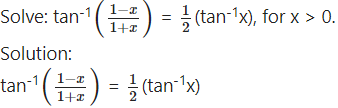

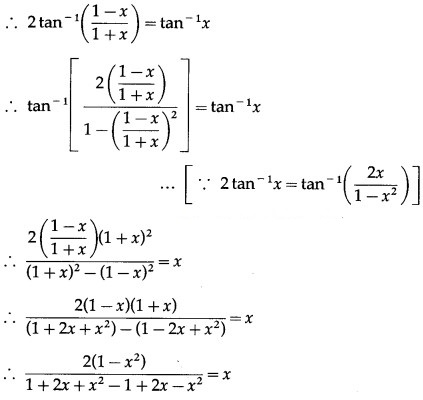
Question 28.
If sin^-1(1 – x) – 2sin^-1x = π/2, then find the value of x.
Solution:
sin^-1(1 – x) – 2sin^-1x = π/2

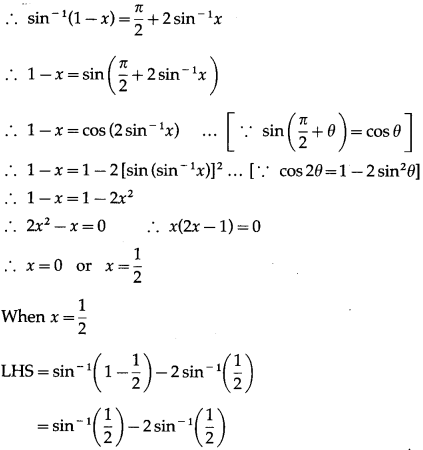
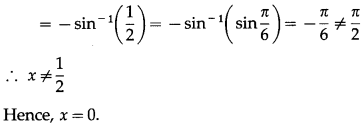
Question 29.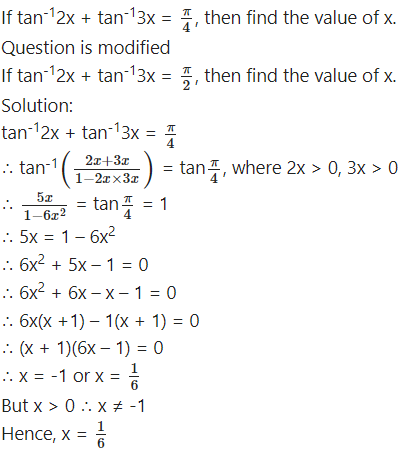

Question 30.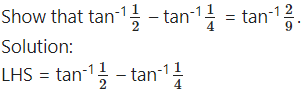

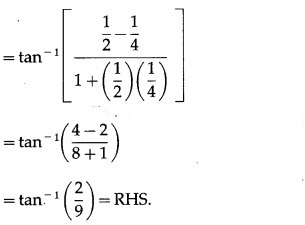
Question 31.

Question 32.

Solution:
We have to show that

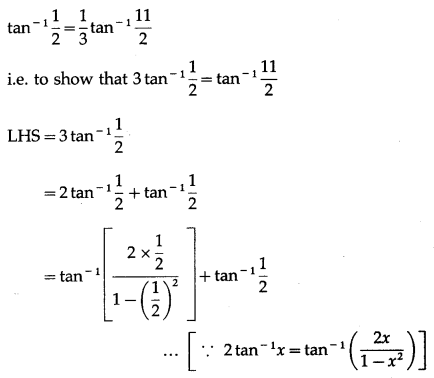
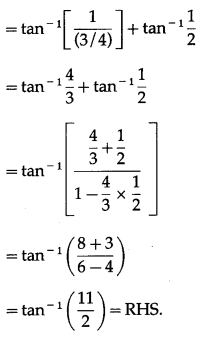
Question 33.

Solution:

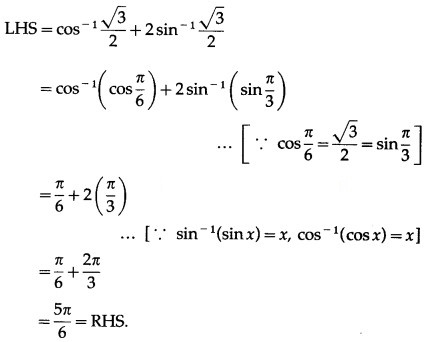
Question 34.

Solution:

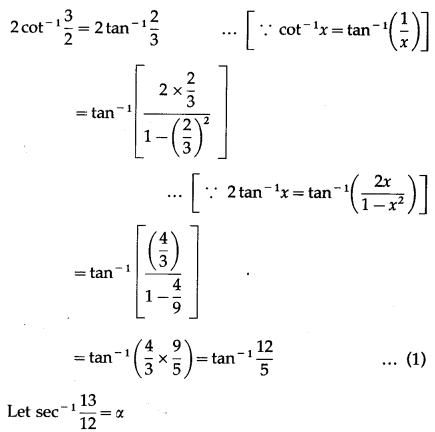
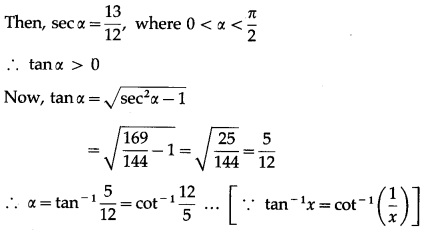
Question 35.
Prove the following :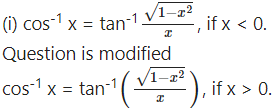

Solution:

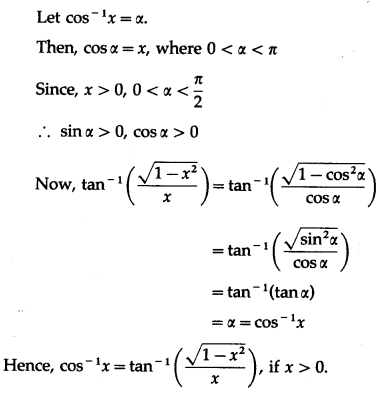
Solution:

Question 36.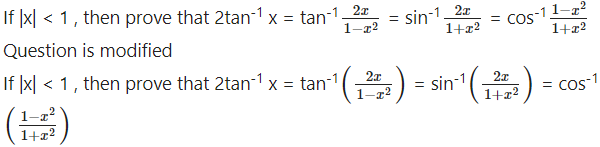

Solution:
Let tan^-1x = y
Then, x = tany

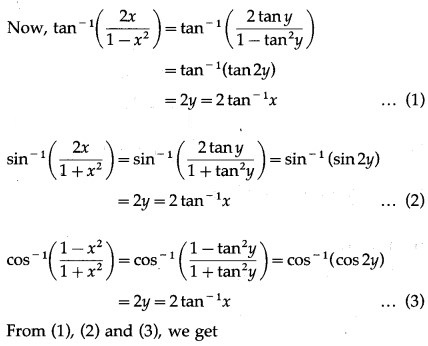
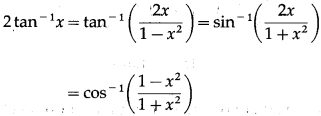
Question 37.

Solution:

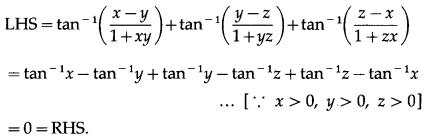
Question 38.
If tan^-1 x + tan^-1 y + tan^-1 z = π/2 then, show that xy + yz + zx = 1
Solution:
tan^-1 x + tan^-1 y + tan^-1 z = π/2

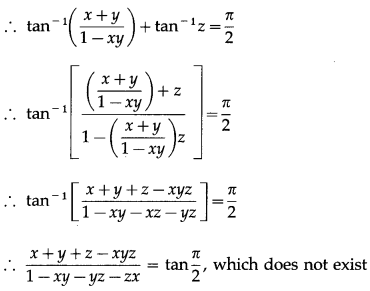
∴ 1 – xy – yz – zx = 0
∴ xy + yz + zx = 1.

Question 39.
If cos^-1 x + cos^-1 y + cos^-1 z = π then show that x² + y² + z² + 2xyz = 1.
Solution:
0 ≤ cos^-1x ≤ π and
cos^-1x + cos^-1y+ cos^-1z = 3π
∴ cos^-1x = π, cos^-1y = π and cos^-1z = π
∴ x = y = z = cosπ = -1
∴ x² + y² + z² + 2xyz
= (-1)² + (-1)² + (-1)² + 2(-1)(-1)(-1)
= 1 + 1 + 1 – 2
= 3 – 2 = 1.