Chapter 4 Determinants and Matrices Ex 4.7
Chapter 4 Determinants and Matrices Ex 4.7
Question 1.
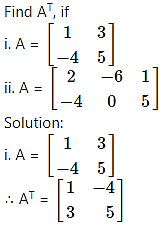

Question 2.
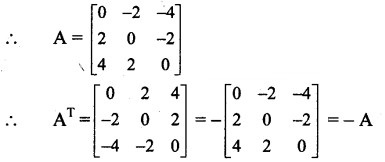
∴ = -A and A = –
∴ A and AT both are skew-symmetric matrices.
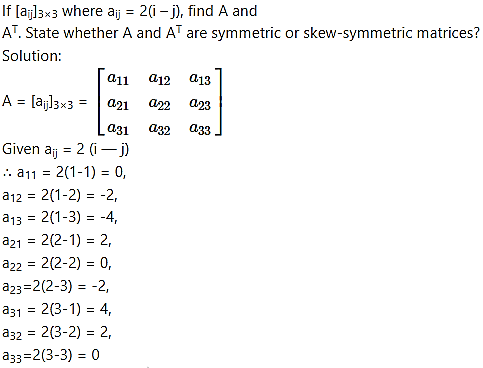
Question 3.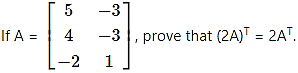
Solution:

From (i) and (ii), we get
(2A = 2
Question 4.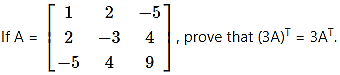
Solution:
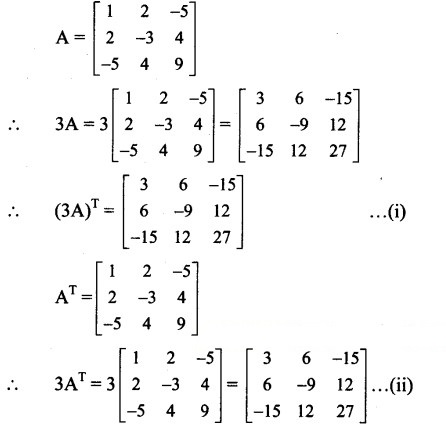
From (i) and (ii), we get
(3A = 3
Question 5.
Solution:
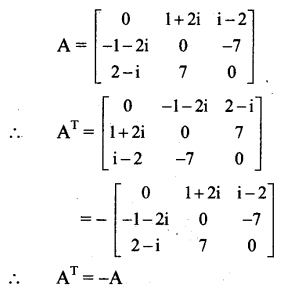
Question 6.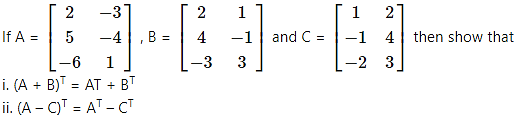
Solution:
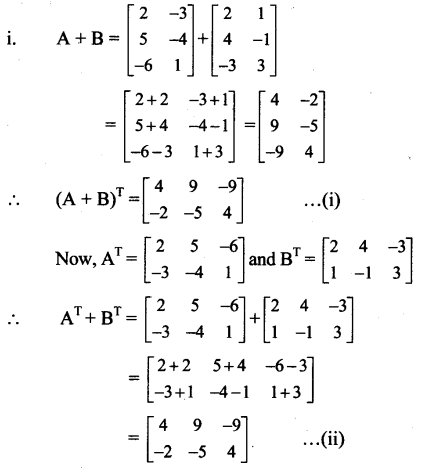
From (i) and (ii), we get
![]()
[Note: The question has been modified.]
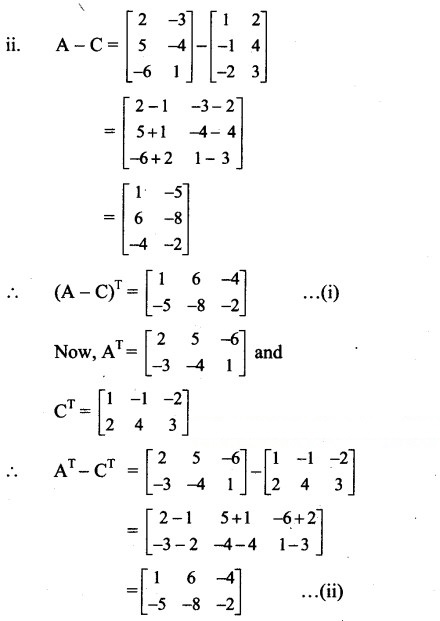
From (i) and (ii), we get
![]()
Question 7.
Solution:
3A – 2B + C = I
∴ C = I + 2B – 3A
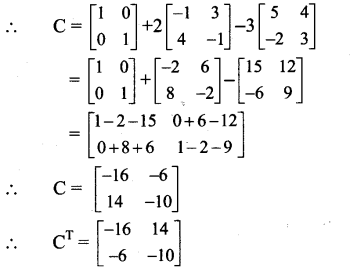
Question 8.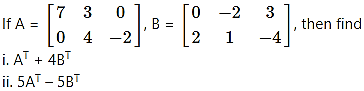
Solution:

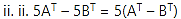
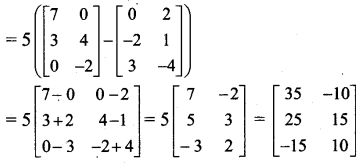
Question 9.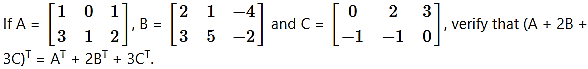
Solution:
A + 2B + 3C
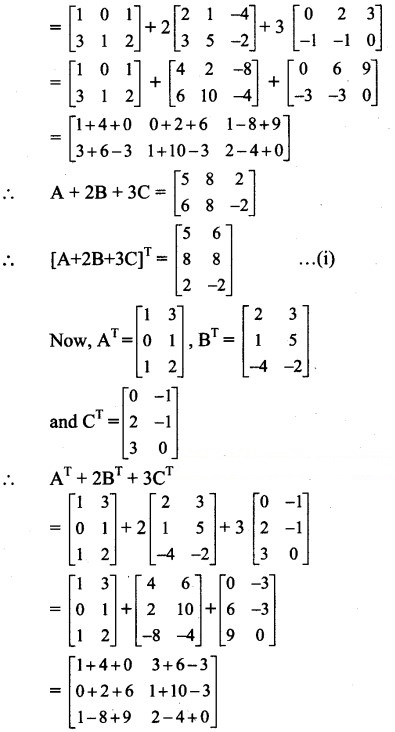

Question 10.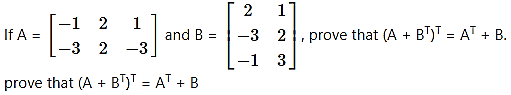
Solution:


From (i) and (ii), we get
![]()
Question 11.
Solution:

![]()
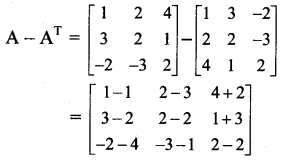


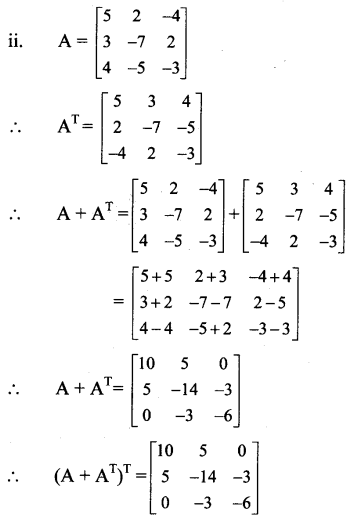
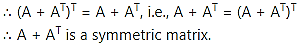
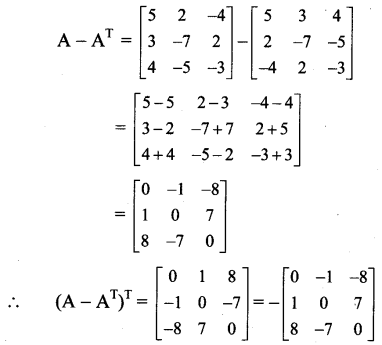
Question 12.
Express the following matrices as the sum of a symmetric and a skew symmetric matrix.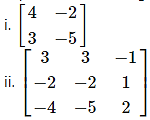
Solution:
A square matrix A can be expressed as the sum of a symmetric and a skew symmetric matrix as
![]()
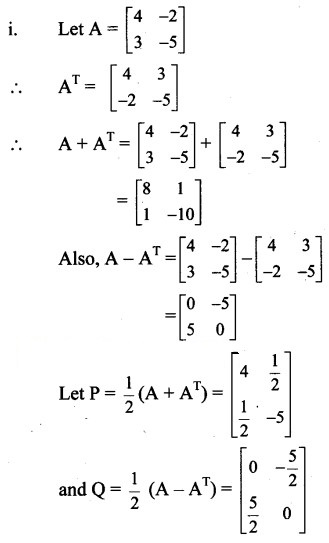
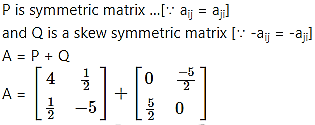
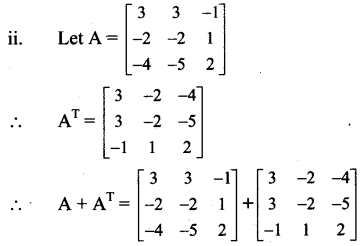
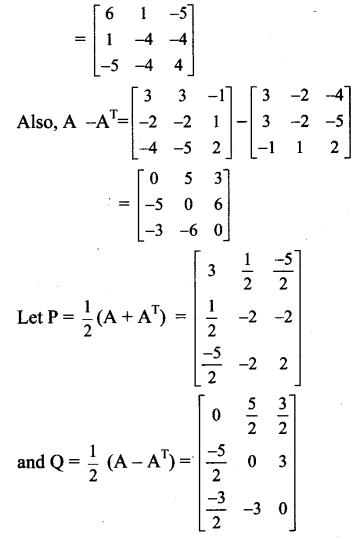
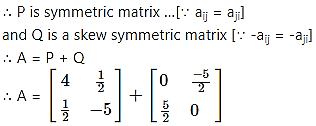
Question 13.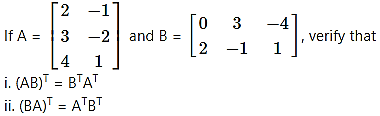
Solution:
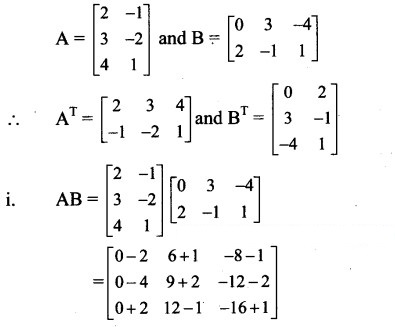
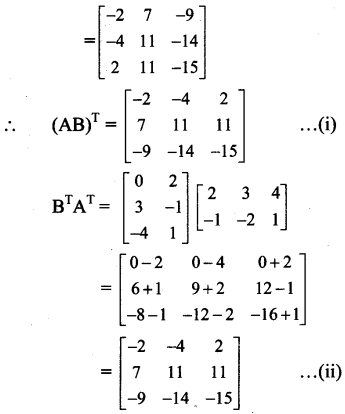
From (i) and (ii), we get
![]()
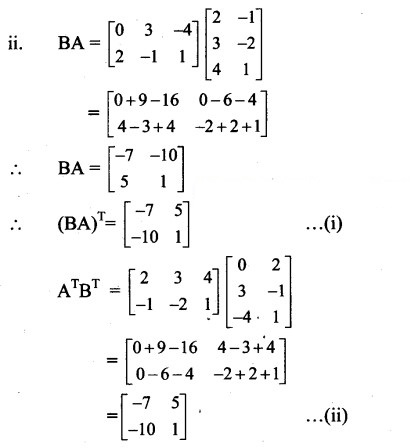
From (i) and (ii) we get
![]()
Question 14.![]()
Solution:


∴ A = I, where I is the unit matrix of order 2.