Chapter 5 Decimal Fractions Set 5.4
Question 1.
Carry out the following divisions.
i. 4.8÷2
ii. 17.5÷5
iii. 20.6÷2
iv. 32.5÷25
Solution:
i. 4.8÷2

ii. 17.5÷5

iii. 20.6÷2

iv. 32.5÷25
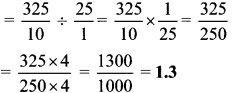
Question 2.
A road is 4 km 800 m long. If trees are planted on both its sides at intervals of 9.6 m, how many trees were planted?
Solution:
Length of road = 4 km 800 m
= 4 × 1000 m + 800 m
= 4000 m + 800 m
= 4800 m
Number of trees on one side = 4800 ÷ 9.6

= 500
∴ Number of trees on both sides = 2 x number of trees on one side
= 2 x 500 = 1000
If the trees are planted at the beginning of the road, then
Total number of trees = 1000 + 2 = 1002
∴ Total number of trees planted is 1000 or 1002.
Question 3.
Pradnya exercises regularly by walking along a circular path on a field. If she walks a distance of 3.825 km in 9 rounds of the path, how much does she walk in one round?
Solution:
Total distance walked in 9 rounds = 3.825 km
∴Distance walked in 1 round = 3.825 4 ÷ 9

= 0.425 km
∴ Total distance walked in 1 round is 0.425 km.
Question 4.
A pharmaceutical manufacturer bought 0.25 quintal of hirada, a medicinal plant, for Rs 9500. What is the cost per quintal of hirada? (1 quintal = 100 kg)
Solution:
Cost of 0.25 quintal of hirada = Rs 9500
∴ Cost of 1 quintal of hirada = 9500 ÷ 0.25
![]()
= Rs 38,000
∴ Cost per quintal of hirada is Rs 38,000.
Intext Questions and Activities
Question 1.
Maths is fun! (Textbook pg. no. 34)
- Consider any three-digit number (say 527).
- Multiply the number by 7. Then multiply the product obtained by 13, and this product by 11.
- The found product is 5,27,527.
Take two or three other numbers. Do the same multiplication and find out how it is done.
Solution:
7 × 13 × 11 = 1001
∴ 527 × 1001 = 527 × (1000+ 1)
= (527 × 1000) + (527 × 1)
= 527000 + 527 = 527527
Thus, when any three-digit number is multiplied with 1001, the product obtained is a six-digit number in which the original three-digit number is written back to back twice.
(Students may consider any other three-digit numbers and verify the property.)